Geometry has become extensively important in terms of understanding mathematical figures and structures. Geometry has various kinds of shapes and figures that are studied to solve complex mathematical problems.
It is highly important to understand and study these figures properly to be able to do mathematical problems related to geometry, including shapes and figures.
Key Takeaways
- A circle is a two-dimensional shape with all points on its boundary equidistant from its center. At the same time, an ellipse is a two-dimensional shape with two axes of symmetry and varying distances from its center.
- Circles are symmetrical and have a constant radius, while ellipses have two different radii and are asymmetrical.
- Circles are used in many applications, including geometry, engineering, and art, while ellipses are used in architecture, design, and astronomy.
Circle vs Ellipse
A circle is a geometric shape with all points lying at an equal distance from its centre, while an ellipse has two focal points and a more elongated form. The shape of an ellipse depends on the distance between its focal points, making it more versatile than a circle in various mathematical applications.
The circle is a geometrical figure which is used to solve problems in mathematics related to geometry. The circle is unique from other geometrical figures as it has the same distance from its centre to any particular point made at the circumference of the circle.
There can be found plenty of examples of circular figures in daily life, such as wheels or bottle caps and so many other instances.
An ellipse is a mathematical figure which is used in geometry to solve geometrical equations related to an ellipse.
An ellipse is more of a curve line made into a plane on both sides. An ellipse may even vary in its size, unlike other geometrical figures.
Comparison Table
| Parameters of Comparison | Circle | Ellipse |
|---|---|---|
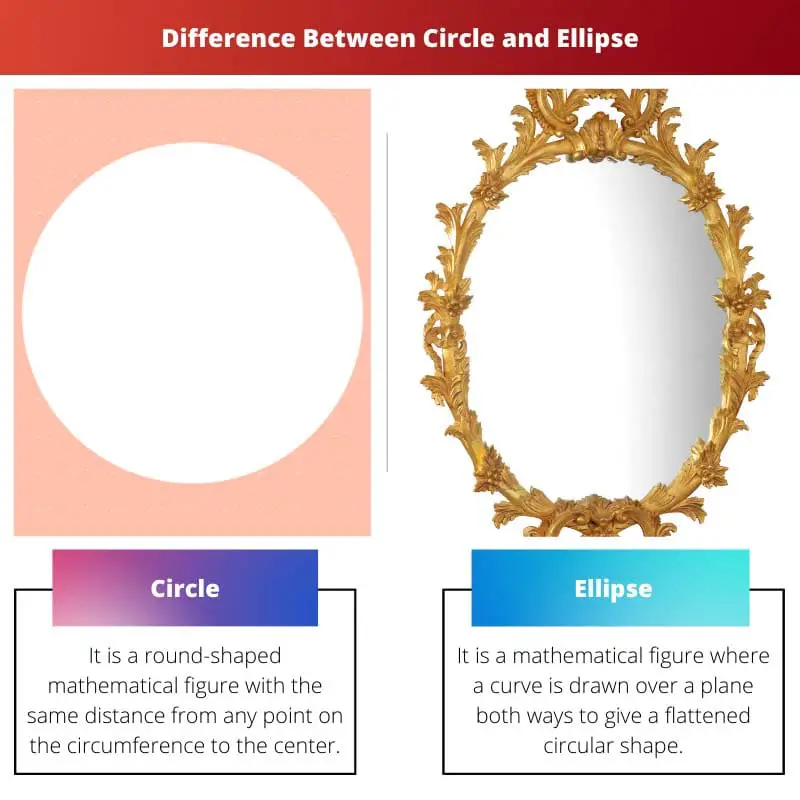
| Definition | It is a round-shaped mathematical figure with the same distance from any point on the circumference to the center. | It is a mathematical figure where a curve is drawn over a plane both ways to give a flattened circular shape. |
| Area | π × r^2 ( r = radius ) | π × a × b |
| Definite shape | It has a definite shape of a round figure. | It may vary from the surface towards a more flattened structure of an ellipse. |
| Distance from center | It has the same distance from the center to any point in the circumference. | It does not have the same distance from the center. |
| Components of figures | One radius at the center. | It has two foci that lie at either end of the ellipse. |
What is Circle?
A circle is a geometrical figure which is more of a round figure shape and is used to solve mathematical equations and problems. It is one of the most common and extensively used mathematical figures, which has its function in geometry.
A circle has a unique feature in that all the points in the circumference of a circle are at an equal distance to the centre. A circle is mostly classified by its shape and the distance from the centre.
The study of geometrical figures has helped over the development of maths and science over time, and the circle is one of such important figures among the others that has also contributed to the study of mathematics.
A circle also has a definite formula to find its radius and other components that are important for the study of geometry. There are multiple examples of circular figures or objects, even in real life, other than mathematical figures.
The main principle with which the formation of a circle works is used in the process of creating circular objects in reality.
These applications and principles are used in mathematics and real life to process these figures, such as the making of wheels in human life.
What is Ellipse?
An ellipse is a geometrical figure that is a curve line drawn in such a manner above a plane line both ways to give it such a flattened circular shape. It is used to solve mathematical equations or problems related to an ellipse.
An ellipse does not have the same distance with the points from that of its circumference to the centre.
The curve is drawn so that the sum of the distance from two different points, known as the foci, while taken from a moving point, is constant.
An ellipse can be made by a cut produced in a cone by an oblique plane without intersecting the base.
An ellipse may vary in shape and is not confined to a particular type of figure. It can be more or less flattened structure or even somewhat closer to the shape of a circle.
Unlike a circle, an ellipse does not have a fixed radius throughout the shape, and it keeps changing.
The most common real-life examples of an ellipse would be the orbits around which the planets are known to revolve around. The astronomical instances can be easily found in real life while studying an ellipse.
Main Differences Between Circle and Ellipse
- A circle has the same distance from any point on the circumference to the centre. An ellipse does not have the same distance from any point to the centre.
- A circle has a fixed shape of a figure even if the viewpoint is moved. In contrast, an ellipse may vary in shape depending on the distance from each focus.
- A circle has a fixed radius that does not change its position. On the other hand, an ellipse does not have a fixed radius throughout the shape of an ellipse.
- The radius of a circle is at the centre, but the two foci of an ellipse lie at either end of an ellipse.
- A circle does not originate from the shape of an ellipse, whereas an ellipse may seem like a flattened circle.
- https://onlinelibrary.wiley.com/doi/abs/10.1901/jeab.1967.10-261
- https://www.sciencedirect.com/science/article/pii/0734189X86902185
I’ve put so much effort writing this blog post to provide value to you. It’ll be very helpful for me, if you consider sharing it on social media or with your friends/family. SHARING IS ♥️
The explanation of the mathematical properties of an ellipse and its application in solving mathematical problems is quite insightful. It shows the significance of ellipses in geometry.
The detailed explanation of what an ellipse is and how it is used to solve mathematical problems provides a comprehensive understanding of the significance of this geometric figure.
The detailed information about the applications and principles of circles and ellipses, as well as the comparison between them, demonstrates their significance and versatility in mathematics and real-life scenarios.
The information about geometrical figures and their mathematical applications is fascinating. It’s intriguing to see how circles and ellipses contribute to the study of mathematics and sciences.
I find it extremely interesting how circles and ellipses are used in various fields such as geometry, engineering, and art. The detailed comparison table is very helpful in understanding the differences between both shapes.
I agree, the applications of circles and ellipses in real life and mathematics are fascinating.
The study of geometrical figures and their mathematical applications has indeed contributed to the development of mathematics and science over time.
The clear explanation of what circles and ellipses are and their usage in mathematical equations is very helpful in understanding their significance in various fields.
The explanation of the formation and application of circles in real life, such as wheels, is intriguing. It’s fascinating to see how mathematical principles are utilized in the physical world.
The discussion on the applications and principles of circles and ellipses in mathematics and real life, such as creating circular objects, is enlightening. It provides a clear understanding of their role in various fields.
The clear definition and detailed explanation of an ellipse, along with its unique properties, make it easier to understand how it is used in mathematical equations.
The detailed information about circles and ellipses, including their definition, components, and applications, provides a comprehensive understanding of these geometric figures and their importance in mathematics and real life. The study of the geometrical principles is truly fascinating.
I agree, the detailed information about circles and ellipses makes it easier to understand their role in mathematics and other fields.
Absolutely, studying geometrical principles and figures contributes to the evolution of mathematics and science.