When a student is learning the subject of algebra, the difference between a function and an equation is always unclear. Equations and Functions are two different topics in the matter of algebra.
Key Takeaways
- An equation is a mathematical statement that equates two expressions, while a function is a mathematical rule that assigns a unique output for every input.
- An equation can have multiple solutions, while a function has only one output for every input.
- Equations are used to solve problems or find unknown values, while functions describe relationships between variables.
Equations vs. Functions
The difference between an equation and a function is that in an equation, a person solving an equation can have either one or two values based on the number of variables they have put into use for solving that equation, and on the other hand, is a function a person will always have solutions based on the Input they have chosen to solve their question.
Equations are a topic used in algebra to solve problems through variables. These equations act as a statement to denote the equality of a variable on both the left and right sides of a word.
Functions are a topic in algebra used by a person to solve problems using variables. When explaining what functions are in algebra, it seems to be a pretty broad topic to be understood.
Comparison Table
| Parameters of Comparison | Equations | Functions |
|---|---|---|
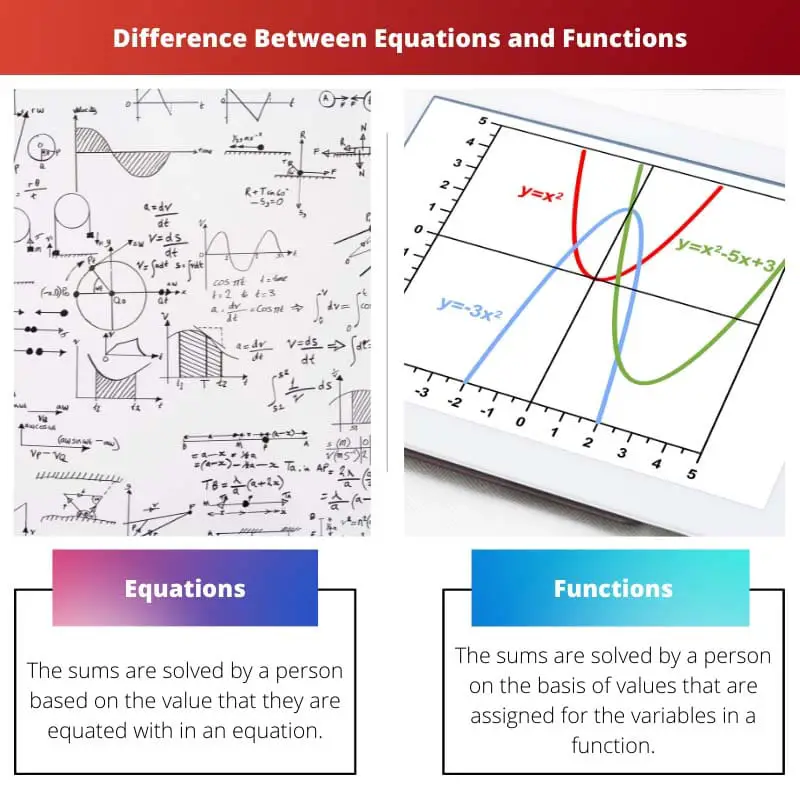
| Solved by | The sums are solved by a person based on the value that they are equated within an equation. | A person solves the sums based on values assigned to a function’s variables. |
| Characteristic | It is a superset of a function. | It is a subset of an equation. |
| Graph | There can be representation on graphs of an equation. | There can sometimes be no representation of graphs of a function. |
| Number of variables | An equation is capable of having more than one value for its variable. | A function cannot have two values for its variable. |
| Points in graph | In a vertical test for equations in a graph, a person can intersect at one or two points at a line. | A person can intersect at multiple points in a graph at a line in a vertical test for functions. |
What are Equations?
Equations are a topic used in algebra to solve problems through variables. These equations act as a statement to denote the equality of a variable on both the left and right sides of a word.
In a function, the right and the left side are always told to be equal. They are always said to have an inverse relationship being unitary by nature when solved. An equation always contains more than one variable.
An equation, in short, means finding the value of a specific variable given in the problem. Following are some examples of the equation.
- 2a + 3a = 15; what are (a) values?
- 4a + 6a = 24; what are (a) values?
What are Functions?
Functions are a topic in algebra used by a person to solve problems using variables. When explaining what functions are in algebra, it seems to be a pretty broad topic to be understood.
In most schools, a function is always taught to a child as a rule which is taken as each set of a member x and mapped to the same value of y on the page.
A letter such as F, a, or g denotes the word function in any algebraic expression. Following are some examples where the problems are solved by using parts.
- F(x) = 3x + 5
- F(g) (x) = 6y+9
Main Differences Between Equations and Functions
- An equation can have more than one value for its variable; conversely, a function cannot have two values for its variable.
- In a vertical test for equations in a graph, a line can intersect at one or two points, and on the other hand, in a vertical test for functions, a line can intersect at multiple points in a graph.
- https://ui.adsabs.harvard.edu/abs/1964RuMaS..19R…1L/abstract
- https://journals.aps.org/prd/abstract/10.1103/PhysRevD.51.5153
I’ve put so much effort writing this blog post to provide value to you. It’ll be very helpful for me, if you consider sharing it on social media or with your friends/family. SHARING IS ♥️
This article efficiently resolves the ambiguity associated with equations and functions.
Indeed, an insightful read that provides coherent differentiation between equations and functions.
An intellectually enriching article that clarifies an confusing academic concept.
The explanation is presented logically and with clarity.
Indeed, it is a commendable explanation. Worth reading.
The logical comparison provided a deeper understanding of the subject.
Informative content. Well-structured comparison between equations and functions.
Yes, it’s insightful and would be very helpful for students.
This article offers deep insights into the dichotomy of equations and functions.
The explanations are intellectually stimulating and add value to the readers’ understanding.
I need more in-depth information to fully appreciate the nuances in the topic.
The author introduces a thought-provoking perspective on equations and functions.
I found the definitions and comparisons very well-explained.
Absolutely. This article provided me with a clearer understanding of the topic.
The article is not comprehensive enough. It could be improved by including more practical examples.
I agree, we need more real-world context for a better understanding.
The content is well-organized and offers a concise comparison of equations and functions.
It is well-written and provides clear distinctions between the two concepts.
The difference between equations and functions is well-articulated in this article.
The examples effectively illustrate the dissimilarities between the two.
The article successfully provides a clear demarcation between equations and functions.
Absolutely useful article for understanding the distinction between equations and functions in algebra.
Yes, it is really informative. I loved the examples provided.