Mathematics is a vast subject. It offers four basic concepts that are addition, subtraction, multiplication, and division.
But a deeper study involves familiarity with concepts like algebra, geometry, trigonometry, mensuration, and many more. The concepts can range from basic to advance and have real-world applications.
Key Takeaways
- Algebra focuses on solving equations and finding unknown values using variables, while trigonometry deals with the relationships between angles and side lengths of triangles.
- Algebra is a fundamental branch of mathematics, whereas trigonometry is a sub-branch of geometry.
- Algebra applies in various fields like physics, engineering, and economics, while trigonometry is crucial for astronomy, navigation, and architecture.
Algebra vs Trigonometry
Algebra is the branch of mathematics that teaches sets, commutative properties, and associative properties, including numbers, points, etc., while Trigonometry is the branch of mathematics that uses sine, cosine, and tangent to solve equations. Algebra has five types, and Trigonometry has two types.
Algebra was introduced before trigonometry in the mathematical world. Algebra requires the manipulation of symbols. It simplifies huge word questions into simple equations and can be solved with various formulas.
Algebra can be further classified into elementary algebra, linear algebra, abstract algebra, and algebraic geometry. Algebra is a combination of variables and constants.
Trigonometry was invented in the 13th century. It involves various functions to find out angles and side relationships with the triangles. It can be further classified into two types, planar and spherical trigonometry.
Comparison Table
| Parameters of comparison | Algebra | Trigonometry |
|---|---|---|
| Discoverer | Discoverer Babylonians invented algebra but Abu Jaafar Mohammad Ibn Mousa Al Khwarizmi worked and perfected the concepts Hipparachus of Nicaea is considered as the father of trigonometry as he was the first mathematician to tabulate the trigonometric functions | Hipparachus of Nicaea is considered as the father of trigonometry as he was the first mathematician to tabulate the trigonometric functions |
| Constituents | Includes integers, calculus, geometry, trigonometry, variables, constants, and other polynomials for equation formation | Includes functions like sine, cosine, tangent, cosecant, secant and cotangent |
| Types | Five types: elementary algebra, abstract algebra, advanced algebra, communicative algebra, and linear algebra | Two types: plane trigonometry and spherical trigonometry |
| Mechanism | To simplify complex word problems into simple polynomial equations | To find out angles of triangles or measurements of sides using various functions |
| Application | Science, medicine, economics, decision making, statistical inferences, graphics, facial recognition technology and etc | Astronomy, navigation, mapmaking, optics, cryptology, oceanography, period functions, medical imaging and etc. |
What is Algebra?
Algebra is derived from the Arabic word “Al-jabr”, meaning reunion of broken parts.
Algebra integrates simple mathematical concepts like integers, natural numbers, whole numbers, factorials and basic properties like commutative, associative, distributive and identity of numbers.
It forms the basis of domains like science, medicine, engineering, economics and many other related fields.
Al-Khwarizmi is known as the father of algebra and referred to Algebra as “The science of restoring and balancing”. A mathematician with expertise and thorough research in Algebra is called an algebraist.
Algebra is a vast subject, has elementary algebra, linear algebra, abstract algebra, universal algebra, and boolean algebra as the sub-parts. Algebra includes calculus, arithmetic, geometry and trigonometry to solve complex problems.
Elementary algebra includes the basics of algebra. The formulation of elementary algebra includes basic arithmetic operators and symbols. Abstract algebra involves sets, binary operations, polynomials, identity elements, inverse elements, associativity and commutativity.
Algebra is utilized functionally in solving various real-life problems like medical diagnosis, decision making, statistical inferences, search engine optimization (SEO), graphics, facial recognition and widely in coding.
Life may not have determined variables like “x” and “y”, but algebra is efficiently valid in different walks of life. Algebra provides adaptability and simplicity in most complex situations.
What is Trigonometry?
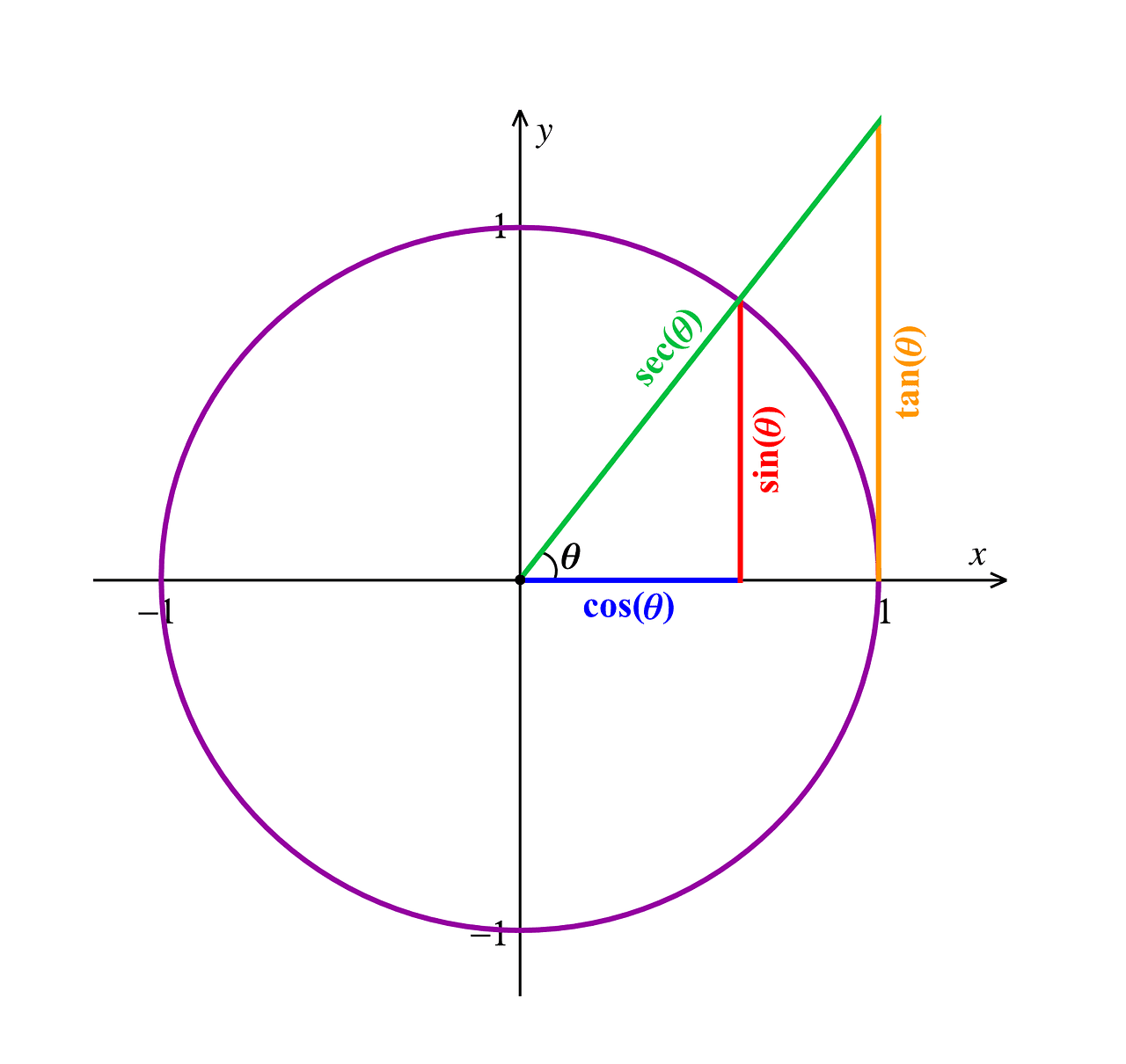
Trigonometry is a concept in Mathematics which consists of angles and sides. The six major functions of angles used in trigonometry are sine, cosine, and tangent, while the reciprocals of these functions are cosecant, secant and cotangent.
These functions have been tabulated to make solving easier. The word trigonometry has been derived from two Greek words, “Trigonon”, meaning triangle and “metron”, meaning to measure.
Historically, Trigonometry was a part of geometry and was declared a separate subject after the 16th century. Hipparachus was the first mathematician to tabulate the values of trigonometric functions.
Trigonometry can be classified into two types: plane trigonometry, which covers angles and distances in one plane and spherical trigonometry, which covers angles and distances in three-dimensional space.
There are various laws that govern the arbitrary triangle states, like the law of sines, the law of cosines and the law of tangents. Identities like Pythagorean identities, Euler’s formula, half-angle identities, the angle sum and difference identities are applied to the subject.
Trigonometry has real-life applications in fields like astronomy, navigation, artillery range evaluation, surveying, mapmaking, periodic functions, optics and acoustics, medical imaging, cryptology and many more.
It was an essential subject during the construction project of pyramids in Egypt. The heliocentric system of Nicolaus and the geocentric system of Ptolemy was based on Trigonometry.
Main Differences Between Algebra and Trigonometry
- Algebra is a concept of maths that deals with variables, constants, equations and rules while trigonometry is a concept of maths that deals with angles of triangles and measurements of sides.
- Algebra uses polynomial equations of constants and variables of “x” and “y” to find a solution, while trigonometry uses the sine, cosine and tangent functions to achieve a solution.
- Al-Khwarizmi is considered the father of Algebra, while Hipparachus of Nicaea is considered the father of Trigonometry.
- Algebra is derived from the Arabic word “Al-jabr “, which means reunion of broken parts, while trigonometry is derived from two Greek words “Trigonon” and “metron”, which means triangle and to measure, respectively.
- Algebra is used in fields like decision making, SEO, graphics while trigonometry is used in fields like astronomy, navigation, optics, acoustics and many other domains.
I’ve put so much effort writing this blog post to provide value to you. It’ll be very helpful for me, if you consider sharing it on social media or with your friends/family. SHARING IS ♥️
