Parallel vs Perpendicular: Difference and Comparison

Geometry has introduced us to several various terms, theories, formulas, definitions, and diagrams. The two most common and widely used terms or descriptions in geometry are parallel and perpendicular.

Both the terms or definitions are very different and unique from each other and don’t share any common similarities.

Key Takeaways

1. Parallel lines never intersect, while perpendicular lines intersect at a 90-degree angle.
2. Parallel lines have the same slope, while perpendicular lines have opposite reciprocal slopes.
3. Parallel and perpendicular lines are commonly used in geometry and are fundamental concepts in mathematics.

Parallel vs Perpendicular

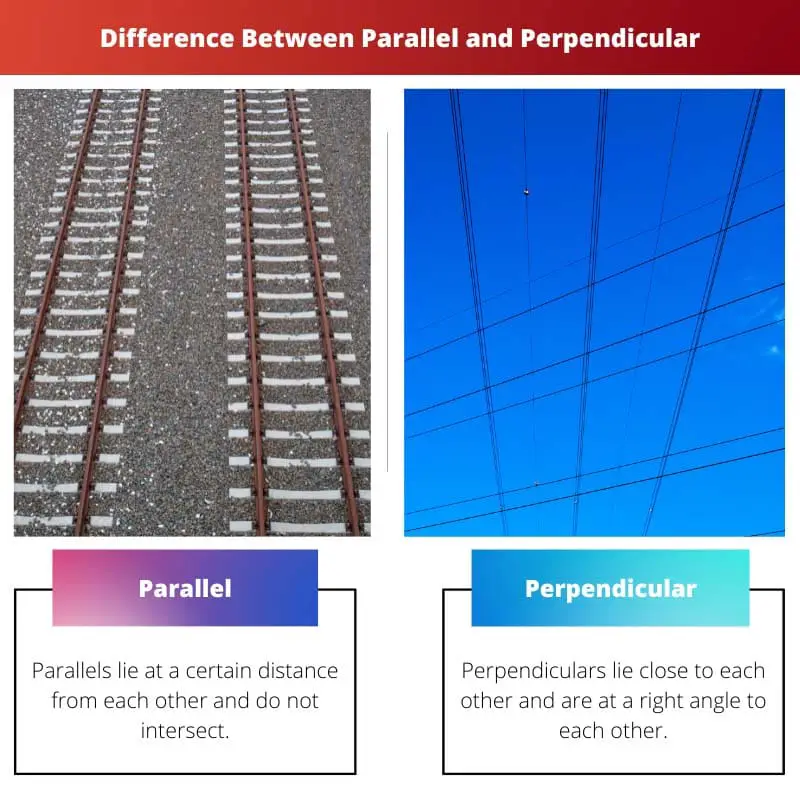
Parallel lines are lines always the same distance apart and never intersect, no matter how far they are extended in both directions. Railroad tracks are parallel lines. Perpendicular lines intersect at a 90-degree angle or a right angle. Vertical lines have slopes that are negative reciprocals of each other.

Parallel lines, curves, or 3 D structures don’t meet at any point. They could refer to either parallel lines on a notepad, opposite sides of a ladder, opposite sides of a road, or opposite sides of a railway track.

These could refer to either lines, boxes, diagrams, or curves.

Perpendicular lines or 3-dimensional figures or curves intersect each other at a particular point. These form right angles with each other.

They refer to either the steps and sides of a ladder, railway track crossing, designs in the window, etc. They have and are represented by a unique symbol and equation.

Comparison Table

Parameters Of Comparison	Parallel	Perpendicular
Significance	Parallels lie at a certain distance from each other and do not intersect.	Perpendiculars lie close to each other and are at a right angle to each other.
Equation	The equation for parallels is y = mx + b.	The equation of perpendiculars is y = mx + a.
Symbol	The symbol, in this case is represented by two lines intersecting each other at a right angle.	Parallel lines or curves always maintain a distance and never intersect.
Intersection	Parallel lines or curves always maintain a distance and hence never intersect each other.	Perpendicular lines or curves intersect each other at a right angle.
Examples	A few examples of Parallels are: •Lines of pages •Telecom Wires	A few examples of Perpendiculars are: •Football Field •Railway Tracks

What is Parallel?

A Parallel could refer to figures, curves, lines, or 3-dimensional boxes. It signifies two lines or curves that run parallel and never intersect.

They are pretty similar to the symbol of an equal sign.

The subject of English defines parallel as an event or happening that occurs at the same time. It refers to events being connected or moving forth in a forward direction.

The English and mathematical terms are quite different from each other.

Parallel lines are represented by two parallel line bars that run similarly. Their symbol is designed as two straight lines at an angle of zero degrees.

The equation y = mx + b represents this term. The “m” remains the same for both parallel lines.

Parallels obey a property called transitive property. According to this property, if line A is parallel to line B and line B is similar to line C, then lines A and C are parallel.

This is one of the most famous and well-known properties of parallel figures

Several examples represent or help us understand parallel lines. These examples are listed below:

• The opposite sides of a constitution are like a rectangle.
• Zebra crossings.
• Staircase.
• Railings.
• The edges of a sidewalk or a roadway.

What is Perpendicular?

Perpendiculars could refer to lines, curves, boxes, or 3-dimensional figures. They run perpendicularly and intersect at a particular point.

The point of Intersection is a right angle for perpendicular figures.

Perpendicularity is described or exhibited in terms of a particular symbol. They also have an equation of their own.

It follows the transitive property, according to which if line X is perpendicular to line Y which is perpendicular to line Z then line X becomes perpendicular to line Z.

Right angles or ninety-degree angles depict perpendicular rays. They are calculated, measured, and constructed with the help of Pythagoras’s theorem.

This theorem and method are used in laying several fields, gardens, and other large areas.

Several examples help us understand perpendicular rays and give us a brief idea of the term. Some of these examples are:

• Designs of a window.
• Football field.
• The crossings of a railway track.
• A house with a wall that lies perpendicular to the floor and ceiling.
• The “plus” sign of a first aid kit or box.

The lines, in this case, are exactly vertical and straight. The letter “T” consists of two lines that lie perpendicular to each other. They lie at a right angle to each other.

Main Differences Between Parallel and Perpendicular

1. Parallel figures run some distance, whereas perpendicular figures run quite close to each other, meeting at a point.
2. The Intersection doesn’t take place in the case of parallel; on the other hand, the Intersection is a common occurrence in the case of perpendiculars.
3. Parallel figures don’t include a 90° angle; on the other hand, perpendicular figures have a right angle.
4. Lines of a roadway refer to parallel dimensions, whereas perpendicular window frames represent perpendiculars.
5. The slopes of parallel diagrams are equal to each other, while on the other hand, the slopes of perpendicular diagrams are unequal.

References

1. https://books.google.com/books/about/Euclid_s_Window.html?id=GHY6VM3NsIwC#v=onepage&q&f=false
2. https://pubs.nctm.org/view/journals/mtms/9/2/article-p84.xml