Las secuencias aritméticas implican una diferencia constante entre términos consecutivos, mientras que las secuencias geométricas implican una relación constante entre términos consecutivos.
Puntos clave
- La secuencia aritmética es una secuencia en la que cada término se obtiene sumando una constante al término anterior.
- La secuencia geométrica es una secuencia en la que cada término se obtiene multiplicando una constante por el término anterior.
- La secuencia aritmética se usa para modelar relaciones lineales, mientras que la secuencia geométrica se usa para modelar relaciones exponenciales.
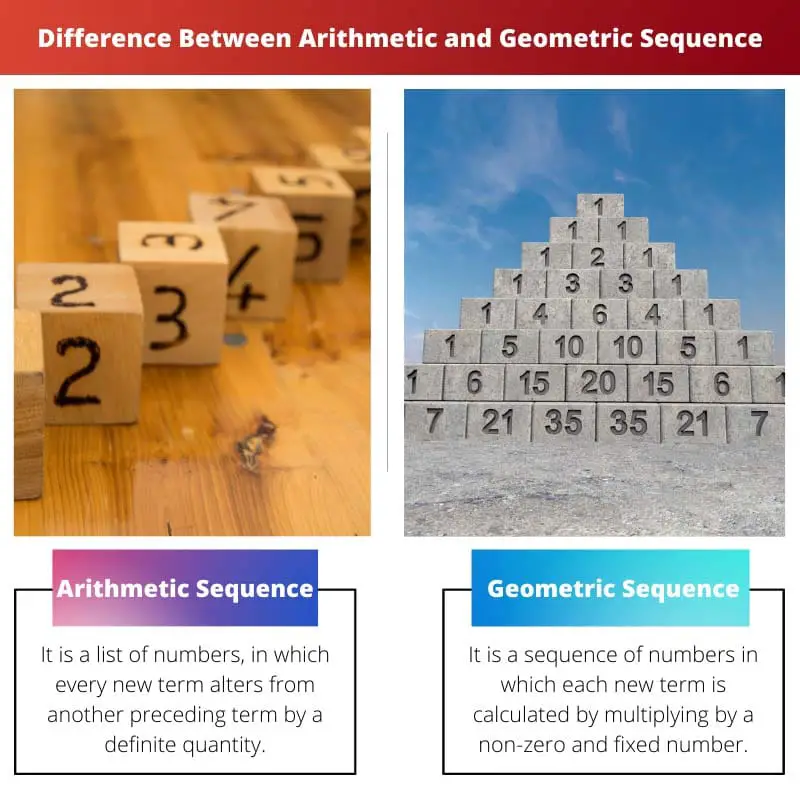
Secuencia aritmética vs geométrica
La variación entre los miembros de una secuencia aritmética es lineal, mientras que la variación en los elementos de la secuencia geométrica es exponencial. La secuencia aritmética infinita diverge; por otro lado, infinitas secuencias geométricas convergen o divergen, según la situación.
La diferencia entre dos términos consecutivos en una secuencia aritmética es común. Por otro lado, la razón de dos términos consecutivos en una secuencia geométrica se conoce como razón estándar.
Tabla de comparación
| Feature | Secuencia aritmética | Secuencia geométrica |
|---|---|---|
| Definición | Una secuencia donde cada término se obtiene sumando un valor constante (diferencia común) al término anterior. | Una secuencia donde cada término se obtiene multiplicando el término anterior por un valor constante (razón común). |
| Fórmula | a_n = a_1 + d(n-1) | a_n = a_1 * r^(n-1) |
| Característica clave | Diferencia constante entre términos. | Relación constante entre términos. |
| Comportamiento | Los términos aumentan o disminuyen en un valor constante. | Los términos aumentan o disminuyen exponencialmente. |
| Suma de los primeros n términos | S_n = n/2 * (a_1 + a_n) | S_n = a_1 * (1-r^n) / (1-r) |
| Ejemplos | 2, 5, 8, 11, 14,… | 2, 6, 18, 54, 162,… |
| Aplicaciones | Cálculos financieros, crecimiento demográfico, física (caída de objetos), teoría musical. | Interés compuesto, decaimiento exponencial, crecimiento poblacional, formas geométricas. |
¿Qué es la secuencia aritmética?
Una secuencia aritmética es una secuencia de números donde cada término es obtenido sumando un valor constante (llamó al Diferencia común) al término anterior. Es un tipo de secuencia específica con comportamiento predecible y aplicaciones en diversos campos.
Aquí hay un desglose de sus características clave:
Definición:
- Una lista ordenada de números donde cada término se obtiene por sumando el mismo número (diferencia común) al término anterior.
Fórmula:
- a_n = a_1 + d(n-1)
- a_n: enésimo término de la secuencia.
- a_1: primer término de la secuencia.
- d: diferencia común.
- n: posición del término en la secuencia.
Característica clave:
- Diferencia común constante: Cada término se diferencia del término anterior por el mismo valor constante, lo que determina la progresión de la secuencia.
comportamiento:
- Progresión lineal: Los términos incrementar o disminuir por un valor constante (d).
- Patrón predecible: Debido a la diferencia constante, los términos de la secuencia son fácilmente predecibles y se pueden calcular mediante la fórmula.
Suma de los primeros n términos:
- S_n = n/2 * (a_1 + a_n)
- S_n: suma de los primeros n términos.
- n: número de términos.
- a_1: primer término.
- a_n: enésimo término.
Ejemplos:
- 2, 5, 8, 11, 14,… (diferencia común de 3)
- -10, -7, -4, -1, 2,… (diferencia común de 3)
- 3, 7, 11, 15, 19,… (diferencia común de 4)
Aplicaciones:
- Finanzas: Calcular intereses compuestos, pagos de préstamos y valores futuros.
- Física: Analizar la caída de objetos, el movimiento de proyectiles y el movimiento armónico simple.
- Teoría musical: Comprender intervalos y escalas.
- Crecimiento de la población: Modelar el crecimiento lineal de la población a lo largo del tiempo.

¿Qué es una sucesión geométrica?
Una secuencia geométrica es una secuencia de números donde cada término es obtenido multiplicando el término anterior por un valor constante (llamó al razón común). Es un tipo de secuencia específico con características distintivas y aplicaciones en numerosos campos.
Aquí hay un desglose de sus características clave:
Definición:
- Una lista ordenada de números donde La relación entre términos se basa en la multiplicación constante..
- Cada término se obtiene por multiplicar el término anterior por un número fijo (razón común).
Fórmula:
- a_n = a_1 * r^(n-1)
- a_n: enésimo término de la secuencia.
- a_1: primer término de la secuencia.
- r: razón común.
- n: posición del término en la secuencia.
Característica clave:
- Razón común constante: La secuencia avanza multiplicando cada término por el mismo valor constante (r), determinando su crecimiento o decrecimiento.
comportamiento:
- Crecimiento o decrecimiento exponencial: Dependiendo del valor de la razón común, los términos de la secuencia pueden aumentar o disminuir exponencialmente.
- Cambio rapido: En comparación con las secuencias aritméticas, las secuencias geométricas experimentan una tasa de cambio más rápida a medida que avanza la secuencia.
Convergencia o divergencia:
- Una secuencia geométrica converge si el valor absoluto de la razón común es menor que 1.
- Divergencia si el valor absoluto de la razón común es mayor o igual a 1.
Suma de los primeros n términos:
- S_n = a_1 * (1-r^n) / (1-r)
- S_n: suma de los primeros n términos.
- n: número de términos.
- a_1: primer término.
- r: razón común.
Ejemplos:
- 2, 6, 18, 54, 162,… (proporción común de 3)
- 1/2, 1/4, 1/8, 1/16, 1/32, … (common ratio of 1/2)
- -3, 9, -27, 81, -243,… (proporción común de -3)
Aplicaciones:
- Finanzas: Cálculo de interés compuesto, modelos de crecimiento exponencial y depreciación.
- Ciencia: Modelado de desintegración radiactiva, crecimiento demográfico con recursos limitados y formas geométricas.
- Teoría musical: Comprender intervalos y logaritmos relacionados con el tono.
- Criptografía: Implementación de algoritmos de cifrado basados en aritmética modular.

Principales diferencias entre secuencias aritméticas y geométricas
- Patrón de progresión:
- Secuencia aritmética: cada término de una secuencia aritmética se obtiene sumando una constante fija (llamada “diferencia común”) al término anterior, lo que da como resultado una progresión lineal.
- Secuencia geométrica: cada término de una secuencia geométrica se obtiene multiplicando el término anterior por una constante fija (llamada “razón común”), lo que da como resultado una progresión exponencial.
- Fórmula:
- Secuencia aritmética: la fórmula general para una secuencia aritmética es an = a1 + (n – 1) * d, donde an representa el enésimo término, a1 es el primer término y d es la diferencia común.
- Secuencia geométrica: la fórmula general para una secuencia geométrica es an = a1 * r^(n – 1), donde an representa el enésimo término, a1 es el primer término y r es la razón común.
- Tasa de Cambio:
- Secuencia aritmética: La tasa de cambio entre términos consecutivos es constante e igual a la diferencia común (d).
- Secuencia geométrica: La tasa de cambio entre términos consecutivos es constante e igual a la razón común (r).
- Ejemplo de progresión:
- Secuencia aritmética: un ejemplo de secuencia aritmética es 2, 4, 6, 8, 10,…, donde la diferencia común (d) es 2.
- Secuencia geométrica: un ejemplo de secuencia geométrica es 3, 6, 12, 24, 48,…, donde la razón común (r) es 2.
- Naturaleza de los términos:
- Secuencia aritmética: los términos en una secuencia aritmética representan cantidades que aumentan o disminuyen en una cantidad fija con cada término.
- Secuencia geométrica: los términos en una secuencia geométrica representan cantidades que crecen o disminuyen en una proporción fija con cada término.
- Suma de términos:
- Secuencia aritmética: La suma de los primeros n términos de una secuencia aritmética se puede calcular usando la fórmula Sn = (n/2) * [2 * a1 + (n – 1) * d], donde Sn es la suma, n es el número de términos, a1 es el primer término y d es la diferencia común.
- Secuencia geométrica: la suma de los primeros n términos de una secuencia geométrica se puede calcular usando la fórmula Sn = (a1 * (1 – r^n)) / (1 – r), donde Sn es la suma, n es el número de términos, a1 es el primer término y r es la razón común.
Última actualización: 11 de diciembre de 2023


Emma Smith tiene una maestría en inglés de Irvine Valley College. Ha sido periodista desde 2002, escribiendo artículos sobre el idioma inglés, deportes y derecho. Lee más sobre mí en ella página de biografía.

La tabla comparativa que enumera las diferencias entre secuencias aritméticas y geométricas es muy informativa y facilita la comprensión de las distinciones entre estos dos tipos de secuencias.
Absolutamente, es una gran referencia para estudiantes y cualquiera que busque comprender las diferencias fundamentales entre secuencias aritméticas y geométricas.
Acordado. La tabla describe claramente las características clave de cada secuencia, lo que facilita la comprensión de los conceptos.
La clara distinción entre los comportamientos y aplicaciones de las secuencias aritméticas y geométricas proporciona información valiosa sobre sus funciones y significado en diversos campos.
No podría estar mas de acuerdo. La publicación resume efectivamente la esencia de ambos tipos de secuencias y sus implicaciones en el mundo real.
La publicación destaca efectivamente las diferencias esenciales entre secuencias aritméticas y geométricas, proporcionando una comprensión clara de sus características y comportamientos únicos.
Absolutamente. La publicación demuestra acertadamente las progresiones lineales y exponenciales contrastantes de estas dos secuencias.
Si bien la explicación de las secuencias aritméticas fue bastante clara, el desglose detallado de las secuencias geométricas proporcionó una visión más profunda de su comportamiento y aplicaciones.
La sección sobre secuencias geométricas me pareció particularmente esclarecedora. Arrojó luz sobre su papel en el crecimiento y la decadencia exponencial, así como sus aplicaciones en el mundo real.
Acordado. Los ejemplos de secuencias geométricas ayudaron a mostrar el rápido cambio y el comportamiento exponencial de estas secuencias.
La explicación completa de las características y comportamientos clave de las secuencias aritméticas y geométricas es muy esclarecedora y sirve como una base excelente para comprender estos tipos de secuencias.
En efecto. Es impresionante cómo la publicación captura efectivamente los matices de ambos tipos de secuencias y sus aplicaciones prácticas.
La sección de aplicaciones arroja luz sobre la relevancia práctica de las secuencias aritméticas y geométricas, mejorando aún más la comprensión de su significado en diferentes dominios.
Absolutamente. Los ejemplos resaltan claramente las amplias aplicaciones de estas secuencias, que van desde cálculos financieros hasta modelos de crecimiento demográfico.
Ciertamente. Las aplicaciones del mundo real proporcionan un contexto valioso para comprender la importancia de estas secuencias en diversos campos.
Las explicaciones completas y los ejemplos ilustrativos brindan una comprensión profunda de los comportamientos y aplicaciones de las secuencias aritméticas y geométricas, lo que las convierte en un recurso valioso tanto para estudiantes como para educadores.
Absolutamente. Las aplicaciones de estas secuencias en diferentes campos están bien presentadas y contribuyen a la claridad general del artículo.
Acordado. La publicación es muy informativa y sirve como una excelente referencia para estudiar las propiedades de estas secuencias.
Las explicaciones detalladas de los comportamientos y aplicaciones de las secuencias aritméticas y geométricas proporcionan una comprensión integral de su importancia en diversos campos.
Definitivamente. Es fascinante ver cómo se aplican estas secuencias en finanzas, física, teoría musical y más.
Absolutamente. Los ejemplos del mundo real ayudan a ilustrar las implicaciones prácticas de estas secuencias.
Esta publicación proporciona una excelente descripción general de las secuencias aritméticas y geométricas y hace un gran trabajo al explicar las características y aplicaciones clave de estas secuencias.
¡Estoy de acuerdo! El desglose de las fórmulas para ambas secuencias es particularmente útil para comprender sus definiciones y comportamientos.
El desglose de las características clave de las secuencias aritméticas y geométricas es claro y conciso, lo que lo convierte en un excelente recurso educativo para estudiantes de matemáticas y campos relacionados.
En efecto. La publicación describe efectivamente los elementos fundamentales de estas secuencias de una manera bien estructurada.