Decimals and fractions are mathematical models that can simplify quite a lot of different types of equations.
Key Takeaways
- Linear functions have a constant rate of change and produce straight lines when graphed.
- Quadratic functions involve a squared term, resulting in a parabolic curve when graphed.
- Both types of functions represent mathematical relationships, but linear functions have a consistent rate of change, while quadratic functions have a variable rate.
Linear vs Quadratic
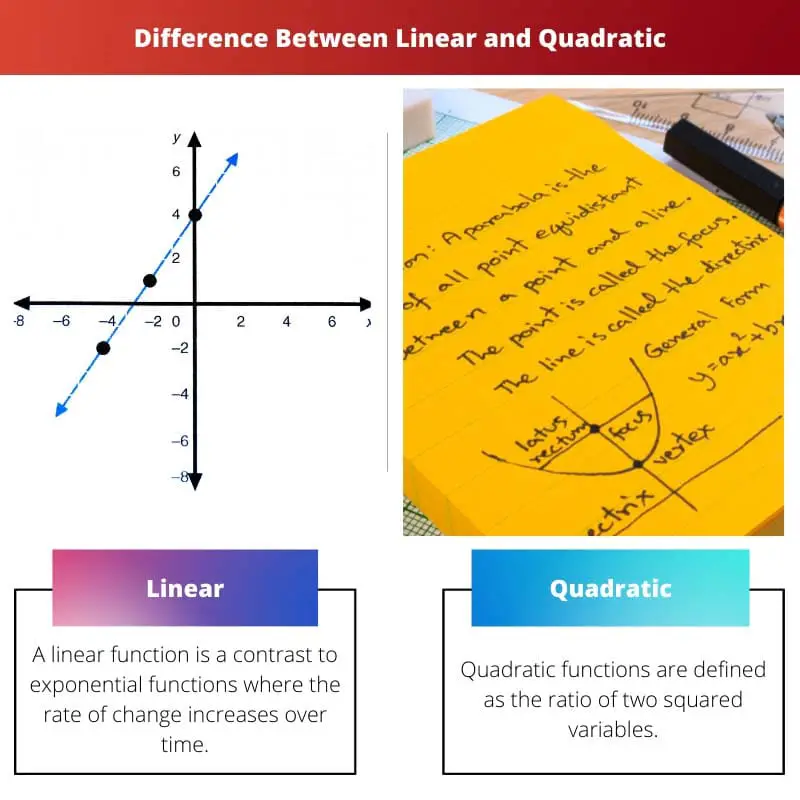
A linear equation is the equation between two variables with a degree of one. On a graph, it is plotted as a straight line. In a linear equation, the rate of change increases with time. A quadratic equation is a polynomial equation with a degree of two. On a graph, it is represented as a parabola.
Moreover, a linear function is in contrast to exponential functions, where the rate of change increases over time.
Quadratic functions are graphically represented as parabolic forms that are seen in physics and mathematics with a degree of two that are written in symbolic and graphical form using x and y coordinates.
Comparison Table
| Parameters of Comparison | Linear | Quadratic |
|---|---|---|
| Defenition | A linear function is a contrast to exponential functions where the rate of change increases over time. | Quadratic functions are defined as the ratio of two squared variables. |
| Degree | Degree of one. | Degree of two. |
| Representation | It is represented as Ax+By+C=0 | It is represented as Ax²+By+c=0 |
| Graphical Representation | Straight line. | Parabola. |
| Example | 1x+4=7, 3x+2=3, 7x=11, x + 3=4 | y = x 2, 5x²+3x+2=0, x² +4x+5=0 |
What is Linear?
Linear are equations that have only one variable of the form ax + by = c. These linear equations can be written in symbolic or graphical form using x and y coordinates where x and y are variables.
The third property is that the left-hand side of an equation equals zero. Some examples of equations are 1x+4=7, 3x+2=3, 5+4x=6 etc.
The first way to minimise the distance between the point of origin and the point on the graph that you wish to find is to use linear functions.
A linear equation is a type of equation that can be written in the form ” a(x+b) = c.” For example, x + 3=4, 3x+2=3, 7x=11 etc or e.g. y=x.
What is Quadratic?
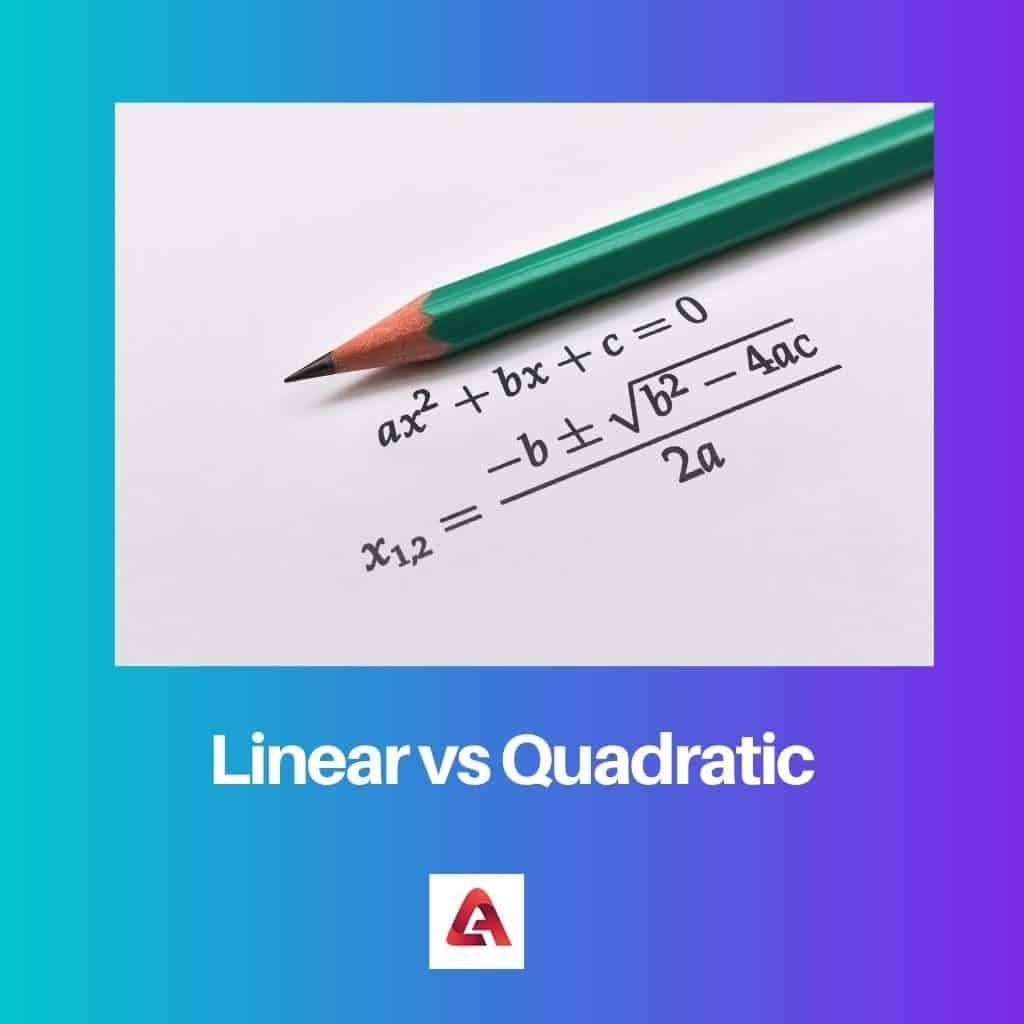
Quadratic functions are quite a bit more difficult than other functions found in mathematics. The only way to solve them is to use a quadratic formula or work it out with a calculator or by hand carefully.
Quadratic functions are commonly seen in physics because they model simple situations that have large changes in the outcome based on small changes in the input.
This is just a Quadratic function example where the Quadratic function bears repeating of the y-axis and x-axis cross at the origin.
The discriminant of a Quadratic function is the square root of the discriminant of the linear function.
Main Differences Between Linear and Quadratic
- Graphical representation of a Linear function is through a Straight line, whereas the Graphical representation of a Quadratic function is through a parabola.
- Examples of Linear functions are 1x+4=7, 3x+2=3, 7x=11, x + 3=4 , whereas Examples of Quadratic functions are y= x 2, 5x²+3x+2=0, x² +4x+5=0.