Line vs Line Segment: Difference and Comparison

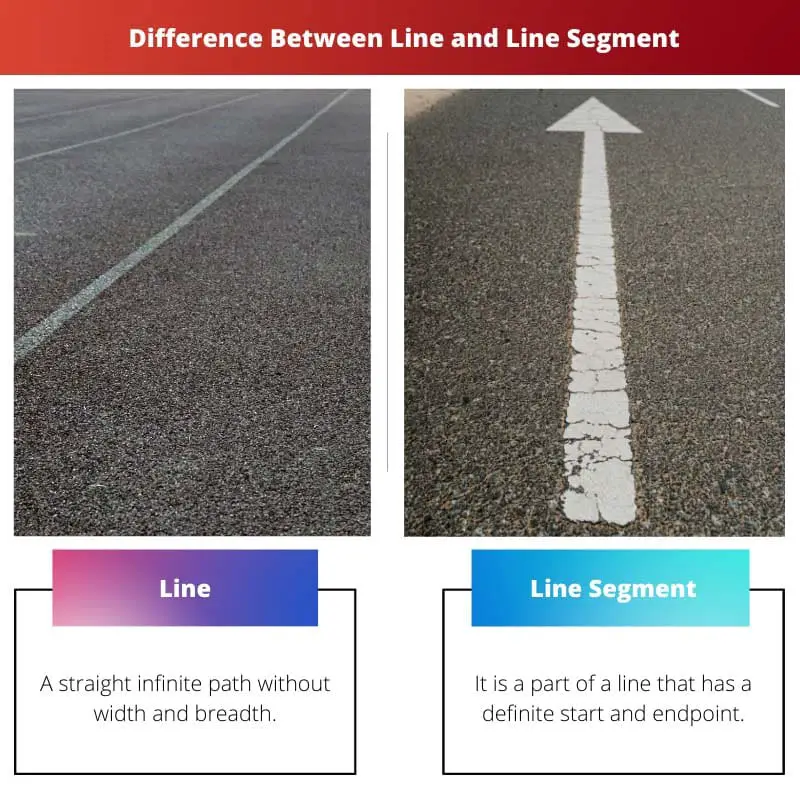
A line is an infinitely extended straight path with no endpoints, continuing indefinitely in both directions. It represents an unbounded geometric concept. In contrast, a line segment is a finite portion of a line with two distinct endpoints. It has a measurable length and does not extend indefinitely like a line.

Key Takeaways

1. A line is a geometric object that extends infinitely in both directions.
2. A line segment is part of a line that has two endpoints.
3. The line has no measurable length, while the line segment has a quantifiable distance.

Line vs Line Segment

A line is a straight, infinitely long geometric figure with no thickness. It can be thought of as a set of points that extends infinitely in both directions. A line segment is a part of a line between two endpoints. Unlike a line, a line segment has a specific length and can be measured.

The extension of the line is shown through arrows at both ends of the line. The arrow is called a ray.

The ray (arrow) merely denotes the infiniteness of the line. A line has no endpoint and can be extended at both ends.

Contrary to the line, a line segment has two definite ends, and the end cannot be extended.

Example: Line –   Shown with arrows

———-à Line

———-à OR <————

Example: Line Segment– shown with two dots

.—————.

Comparison Table

Feature	Line	Line Segment
Definition	A straight, one-dimensional figure that extends infinitely in both directions.	A straight, one-dimensional figure with two distinct endpoints.
Endpoints	No endpoints	Two defined endpoints
Length	Infinite	Has a definite length, measured by the distance between its endpoints.
Symbol	Represented by two letters (e.g., AB), an arrow above a single letter (e.g., AB), or simply the letter itself (e.g., l).	Represented by a line segment with a bar above the letters denoting the endpoints (e.g., AB).
Drawing	Often depicted with arrows on both ends to indicate its infinite extension.	Drawn as a straight line with two distinct points marking its beginning and end.
Examples	The edge of a ruler, the horizon line in a painting.	A segment of a road, a side of a square.

What is a Line?

A line segment is a straight path that connects two specific points, known as endpoints, in a given space. Unlike a line, which extends infinitely in both directions, a line segment has a finite length delimited by its endpoints. These endpoints determine the boundary of the segment, defining its extent within the space.

Characteristics

1. Finite Length: Unlike a line, which has infinite length, a line segment possesses a measurable, finite length determined by the distance between its two endpoints. This characteristic makes line segments suitable for representing distances and measuring spatial quantities in various contexts.
2. Distinct Endpoints: Every line segment has two endpoints, which are unique points that mark its boundaries. These endpoints serve as reference points for defining the extent of the segment and distinguishing it from neighboring geometric entities.
3. Straight Path: A line segment follows a straight path between its endpoints, maintaining a constant direction and distance throughout its length. This characteristic ensures that the segment remains geometrically consistent, facilitating precise calculations and geometric constructions.
4. Exclusivity: The points lying on a line segment are confined within its boundaries, excluding the endpoints themselves. This exclusivity distinguishes the interior of the segment from its endpoints, enabling clear delineation of geometric regions and precise analysis of spatial relationships.

What is a Line Segment?

A line segment is a straight path that connects two distinct points in space. Unlike a line, which extends indefinitely in both directions, a line segment has a defined length bounded by its two endpoints. These endpoints mark the limits of the segment, defining its extent within the geometric space.

Characteristics of Line Segments

1. Distinct Endpoints: Every line segment possesses two unique endpoints that delineate its boundaries. These endpoints are specific points in space, serving as reference markers for the beginning and end of the segment. They are essential for defining the length and position of the segment within the overall geometry.
2. Finite Length: Unlike a line, which has infinite length, a line segment has a measurable, finite length. This length is determined by the distance between its two endpoints, providing a quantitative measure of the segment’s extent. The finite nature of line segments makes them suitable for representing distances and measuring spatial quantities accurately.
3. Straight Path: A line segment follows a straight path between its endpoints, maintaining a consistent direction and distance throughout its length. This straightness ensures geometric integrity and facilitates precise calculations and constructions involving the segment. The straight path property distinguishes line segments from curved paths, such as arcs or circles.
4. Exclusivity of Points: The points lying on a line segment are confined within its boundaries, excluding the endpoints themselves. This exclusivity ensures that the interior of the segment comprises only the points directly connected by the straight path between the endpoints. It allows for clear delineation of geometric regions and facilitates rigorous analysis of spatial relationships.

Significance and Applications

Line segments are indispensable in geometry, mathematics, and various practical fields. They serve as foundational elements in geometric constructions, providing the basis for drawing shapes, measuring distances, and defining geometric relationships. Line segments are extensively used in fields such as engineering, architecture, physics, and computer graphics for modeling spatial structures, calculating dimensions, and simulating physical phenomena.

Main Differences Between Line and Line Segments

• Definition:
  • A line is an infinitely extended straight path with no endpoints.
  • A line segment is a finite portion of a line with two distinct endpoints.
• Length:
  • A line has infinite length, extending indefinitely in both directions.
  • A line segment has a finite, measurable length delimited by its endpoints.
• Endpoints:
  • A line has no endpoints; it continues indefinitely in both directions.
  • A line segment has two distinct endpoints that mark its boundaries.
• Geometric Representation:
  • A line is represented by a straight, unbounded path without any breaks.
  • A line segment is represented by a straight path between two endpoints, with a finite length.
• Usage:
  • Lines are used to represent directions, boundaries, or rays in geometry.
  • Line segments are commonly used for measuring distances, defining shapes, and constructing geometric figures.