Maths is a study of all the world’s numbers, theorems, and formulas, which remains standard worldwide.
Maths has developed a lot, and today it is used in every aspect of our life, from cutting a perfect square of wood to making a stand.
Using maths to develop maps of a particular building to turn it into beautiful skyscrapers, maths has played a significant role in our lives.
Studying numbers and maths helps us build buildings and make perfect food using the exact grams and milligrams to prepare food.
The area has always helped determine the ideal planning of structures and buildings; if a concept like an area had never existed, city planning and house planning wouldn’t have been possible.
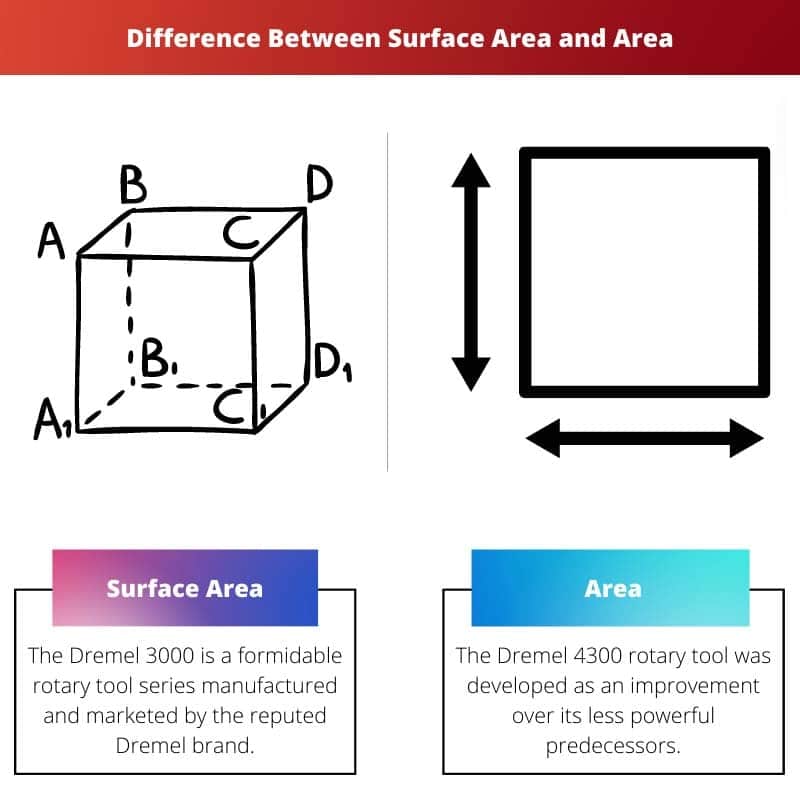
Area and Surface area are the most used terminologies, even taught in school. It is changeable, but there is a vast difference.
Key Takeaways
- Area refers to the measurement of a two-dimensional shape, while surface area refers to the measurement of the total area of a three-dimensional object’s surfaces.
- The area is measured in square units, while surface area is measured in square or cubic units, depending on the object.
- The area can be calculated for any two-dimensional shape, while surface area can only be calculated for three-dimensional objects such as cubes, cylinders, or spheres.
Surface Area vs. Area
The difference between area and surface area is that area is calculated for the 2D figures, meaning the area is used to calculate the area occupied by the figure calculated in square units, the SI unit of measurement. Surface area is used for calculating the area of all the 3D shapes, including all the sides, top, and bottom of any figure; we add all the surfaces to get the surface area.
For example, in a rectangle, when we calculate area, we multiply the length and the breadth(L×B), but to calculate surface area, we add all four surfaces, double the measurements, and multiply (2LH×2LW×2WH)
Comparison Table
| Parameters of Comparison | Area | Surface Area |
|---|---|---|
| Meaning | Used for calculating the space occupied by a 2D figure, the number of square units occupied ex-square. | Used for calculating the area occupied by the 3D figures, being a 2D figure on paper, we add all the surfaces giving the actual space occupied. |
| Extensions | None | TSA- Total surface area LSA- Lateral surface area CSA- curved surface area |
| Formulas | A square is a 2D object, so we calculate it by multiplying the base with the height (B×H) | The cube is a 3D figure of the square. We calculate it by multiplying the edge with six (a2×6) |
| Area of Focus | While calculating the area, the focus remains on one site. | While calculating the surface area, the focus is on all areas of the face of the shape. |
| Used For | It helps in calculating the square units occupied by the 2D object. | It helps in calculating the actual area occupied by the 3D figure. |
| Type of figures | It is used for plain figures like rectangles, squares, and circles. | It is used for solid figures like cubes, cuboids, and pyramids. |
What is Area?
The area is defined as the square units occupied by a two-dimensional shape. The area calculates the occupation of two-dimensional figures like rectangles and square circles.
A straightforward example to understand the area is if we want to paint a wall in a house, we should know the exact size of the border: the length and the breadth, to see the cost of painting and the amount of paint required.
Area not only plays a vital role in modern mathematics as it is used in geometry and calculus.
The area is used to know the exact size and to construct a building or a house.
The standard international unit of area is a meter square which is 1 meter multiplied by 1 meter resulting in a whole meter square.
A rectangle with different sides says a length of 4 meters and a width of meters. The area calculated is 8 meters square, equivalent to 8 million square millimeters.
What is Surface Area?
The surface area measures the space occupied by a 3D or a definite shape.
Since the face of a three-dimensional figure is a two-dimensional figure, calculate the area by adding all the surfaces of an object.
The surface area also has extensions. The first one is the curved surface area, which includes the area of all the curved surfaces.
The second one is the lateral surface area, which includes all the surfaces but not the top and bottom areas.
The third extension is the total surface area, including all the surfaces and the top and bottom.
Surface area is used to calculate all the objects in real life, helping us know the actual space occupied by an item.
For example, if we are building a wall, we need to calculate the length, breadth, and width to know the actual area occupied and the total area covered by the wall.
The surface area helps to calculate the size of reliable figures like cubes, cuboid pyramids, etc.
While calculating the surface area, we take an instance of all the plane figures, calculate the actual area, and then multiply it to get the result.
Main Differences Between Surface Area and The Area
- The area is used for calculating the area occupied by a 2D figure like a rectangle, square, or circle. In contrast, the surface area is used for calculating the area occupied by a 3D figure like a cube, cuboid, etc.
- The area has no further extensions, whereas the Surface area has three extensions: total surface area, lateral surface area, and curved surface area.
- While calculating the area of, say, a rectangle being a 2D figure, only length and breath will be calculated; therefore, while calculating area, only the sides are put in focus, whereas while calculating the surface area, say cuboid, all sides, that is, length, breadth, and height, will be taken into focus therefore while calculating surface area all dimensions are calculated.
- For instance, the area can be calculated of the wall to check the amount of paint required to paint the wall. In contrast, the surface area is used for items like a cabinet that will be put in a room whose length, width, and height will be calculated to know the area occupied.
- Hence, area calculates the square meter of any area, whereas surface area calculates the exposed dimensions and area of occupancy.
I’ve put so much effort writing this blog post to provide value to you. It’ll be very helpful for me, if you consider sharing it on social media or with your friends/family. SHARING IS ♥️
A concise yet informative guide to understanding the concepts of area and surface area in mathematics.
The article provides a comprehensive analysis of area and surface area, elucidating their significance in different mathematical contexts.
The detailed comparison table efficiently highlights the key differences between area and surface area, making it easier for readers to grasp the concepts.
This article has provided a well-articulated explanation of area and surface area, offering clear examples to elucidate the concepts.
This article presents a comprehensive overview of mathematical concepts in the real world. It provides an insightful perspective on how mathematics is interconnected with various aspects of life.
An excellent portrayal of the significance of mathematical concepts in our everyday life. The detailed comparison between area and surface area provides a great understanding of their applications and differences.
Absolutely, the practical examples and clear explanations make it easier for readers to comprehend the topics.
While the article focuses on the fundamental concepts of area and surface area, it lacks discussion on advanced applications of these mathematical principles.
I agree, it would be beneficial to explore further into the applications of area and surface area in engineering, physics, and other fields.
The article has successfully presented complex mathematical concepts in a simplified manner, fostering a better understanding of the subject.
This article effectively outlines the distinction between area and surface area. However, the mathematical formulas could have been presented more systematically for better clarity and comprehension.
Indeed, a more structured approach to presenting the mathematical formulas would enhance the educational value of the article.
The practical applications of area and surface area are effectively depicted, demonstrating the relevance of mathematics in various fields.